Ergun Akleman on Space Filling Structures

A space filling shape is a cellular structure whose replicas together can fill all of space watertight, i.e. without having any voids between them, or equivalently, it is a cellular structure that can be used to generate a tessellation of space [Williams1979]. 2D tessellations and 2D space filling shapes are relatively well-understood. However, problems related to 3D tessellations and space-filling shapes are still interesting and have applications in a wide range of areas from chemistry and biology to engineering and architecture.
A well-known anecdote demonstrates the difficulty of 3D tessellations is that Aristotle claimed that the tetrahedron can fill space and many people tried to prove his claim despite the fact that the cube is the only space filling Platonic solid. Great Russian Mathematician Delaunay was the first to develop a method to investigate space filling polyhedral using symmetry operations on points and Voronoi diagrams to produce space filling poyhedra, which he called Stereohedra [Delaunay1961].
We, now, know that there are only eight space-filling convex polyhedra and only five of them have regular faces, namely the triangular prism, hexagonal prism, cube, truncated octahedron and Johnson solid gyrobifastigium [Williams1979]. It is also interesting that five of these eight space-filling shapes are "primary" parallelohedra, namely cube, hexagonal prism, rhombic dodecahedron, elongated dodecahedron, and truncated octahedron.
Gomez-G’alvez et al. recently observed a generalization of parallelohedra commonly exist in epithelial cells in the formation of thin skin layers [gomez2018]. These shapes, they call "scutoids", have both polyhedral and parallelohedral forms by providing a natural solution to three-dimensional packing of epithelial cells.
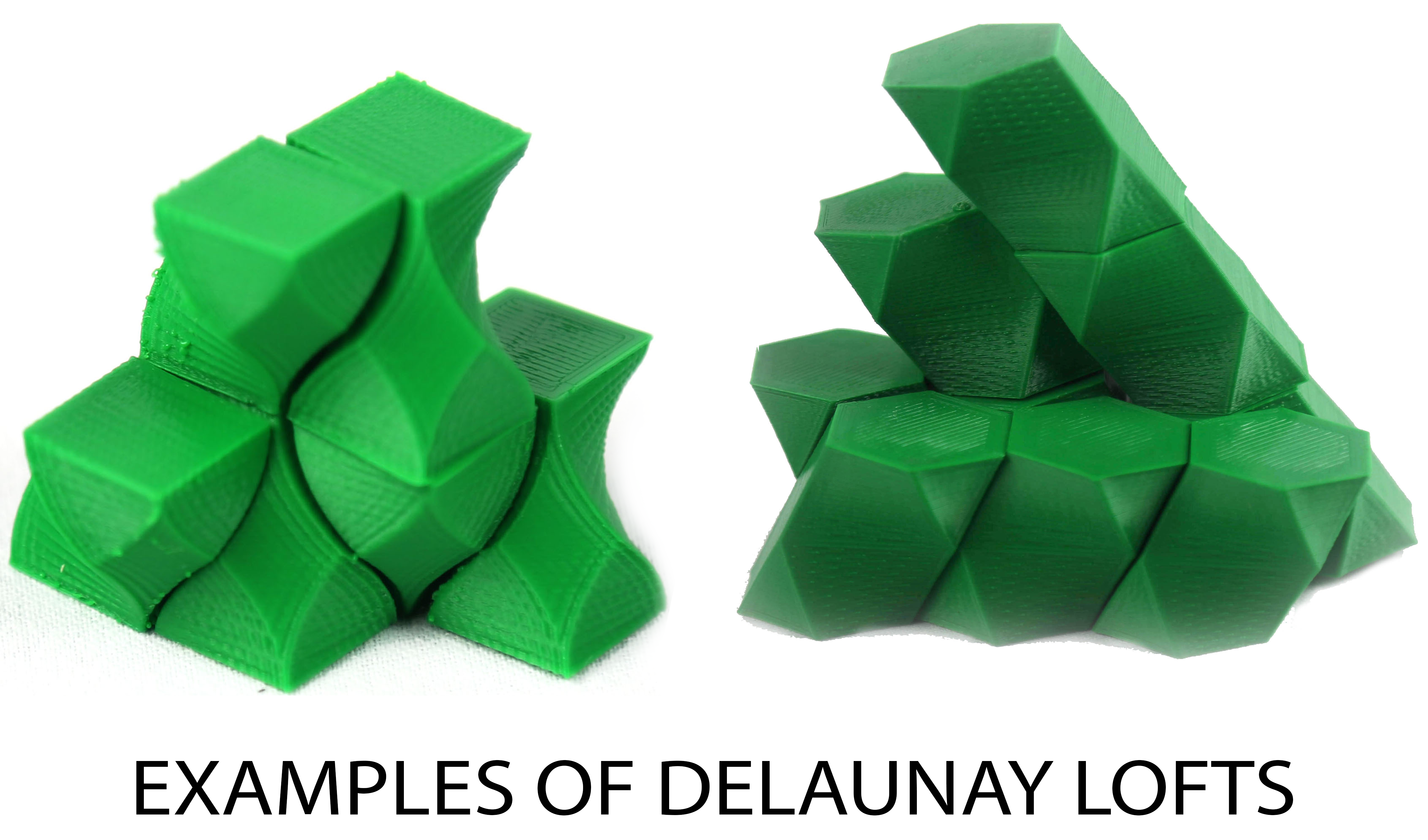 Inspired by Scutoids, we have recently developed an approach to construct and eventually design a new class of space filling shapes in 3-space. Our space filling structures have curved edges and curved faces such non-planar pentagons or hexagons. Our approach is also in-sync with Delaunay's original intention to produce space-filling structures using Voronoi diagrams. Our approach is based on interpolation a stack of planar tiles whose dual tilings are Delaunay diagrams. We construct control curves that interpolate one Delaunay vertex of each planar tile. Voronoi decomposition of the volume using these control curves as Voronoi sites gives us lofted interpolation of original faces. This, combined with the use of wallpaper symmetries allows for the design of the new class of space filling structures in 3-space, , which we call Delaunay Lofts.
Inspired by Scutoids, we have recently developed an approach to construct and eventually design a new class of space filling shapes in 3-space. Our space filling structures have curved edges and curved faces such non-planar pentagons or hexagons. Our approach is also in-sync with Delaunay's original intention to produce space-filling structures using Voronoi diagrams. Our approach is based on interpolation a stack of planar tiles whose dual tilings are Delaunay diagrams. We construct control curves that interpolate one Delaunay vertex of each planar tile. Voronoi decomposition of the volume using these control curves as Voronoi sites gives us lofted interpolation of original faces. This, combined with the use of wallpaper symmetries allows for the design of the new class of space filling structures in 3-space, , which we call Delaunay Lofts.
References:
[Delaunay1961] Boris Nikolaevich Delaunay and Nina Nikolaevna Sandakova. Theory of stereohedra. Trudy Matematicheskogo Instituta imeni VA Steklova, 64:28–51, 1961.
[Gomez-Galvez 2018] Pedro Gomez-Galvez, Pablo Vicente-Munuera, Antonio Tagua, Cristina Forja, Ana M Castro, Marta Letran, Andrea Valencia-Exposito, Clara Grima, Marina Bermudez-Gallardo, Oscar Serrano-Perez-Higueras, et al. Scutoids are a geometrical solution to three-dimensional packing of epithelia. Nature communications , 9(1):2960, 2018
[Subramanian 2019] Subramanian, Sai Ganesh, Mathew Eng, Vinayak R. Krishnamurthy, and Ergun Akleman. "Delaunay Lofts: A Biologically Inspired Approach for Modeling Space Filling Modular Structures." Computers & Graphics (2019).
[Williams 1979] Robert Williams. The geometrical foundation of natural structure: A source book of design. Dover New York, 1979.